Why are there fewer board games with a triangular grid?

Question

Many games have a rectangular grid, some even use hexagonal but it's quite rare to see triangles. I have heard the argument that hexagons confuse players. Is that true? Is there any evidence to suggest it?

My inner game designer thinks that hexagonal games have more choices. So a casual player might be paralyzed by so many options compared with what he "knows as normal". So a 'hardcore' player will love it, again for the extra choices. If that's true, and not just a rationalization I made up, then will a triangular board feel constricted to both demographics?

Is that line of reasoning sound? Is there something I'm missing?

Answer 1

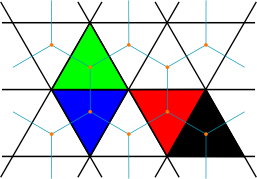
A hexagonal grid and a triangular grid are duals of each other; that is, if you put a dot in the center of each hexagon, and connect them to each adjacent one, then you get a triangular grid:

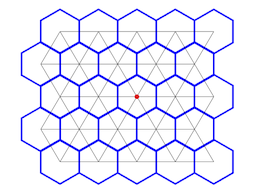
Thus, playing on the vertices of a triangular grid is equivalent to playing in the spaces on a hexagonal grid, and likewise playing on the vertices of a hexagonal grid is equivalent to playing in the spaces on a triangular grid. An example of this is the game Hex, so named because of the hexagonal grid it is played on; however, you can also play it on a triangular grid by playing it like Go, on the vertices instead of the spaces.

One of the big difference between playing (in the spaces) on a hexagonal grid and a triangular grid is that in a hexagonal grid, each space is connected to six spaces around it; in a triangular grid, each space is connected to only three. This can significantly limit the number of options, if for instance you are only allowed to move to adjacent spaces, or only are allowed to make connections to adjacent spaces. A square grid gives you four connections, or eight if you can move or connect diagonally. A hexagonal grid gives you six. In many game designs, only have three moves or connections available is likely to be fairly limiting, though it may be possible to do something interesting with that.

There are games played with triangular grids. One example that comes to mind is Blokus Trigon. This is an interesting case, as each piece consists of triangles joined by their edges, but you join your pieces together by their corners, with the restriction that each piece must touch another of the same color on a corner but not on an edge. In the traditional square version of Blokus, this means that only two of the pieces which touch at a given intersection may be the same color; while in the triangular version, you have 6 spaces around each intersection, and thus can fit three pieces of the same color in. In this way, it manages to take advantage of both the triangular and hexagonal properties of the triangular grid.

Another example of using triangular and hexagonal boards is attempting to play Go on both. Xah Lee has some notes on how that works. As Go is played on the intersections, not in the spaces, each piece connects to only three others on the hexagonal board, and thus each piece has only three liberties, while on the triangular board, each space connects to six others. In the hexagonal version, only three spaces means that each piece is very vulnerable to capture; the game is very unstable. In the triangular version, each piece has lots of liberties, and is quite hard to capture. It sounds like for Go, 4 connections really is the ideal balance for just enough risk to have good tactical battles, while enough stability to be able to form live groups and be able to think more about strategy.

In summary, triangular grids are used occasionally, but I believe that in many cases they provide too few options or connections to provide interesting strategic play. However, if you're interested in designing games, perhaps you should take that as a design challenge; try to design a game that takes good advantages of the limited number of options a triangular grid gives you.

Image credits

• Dual of hexagonal grid by Antal Spector-Zabusky of StackOverflow. Licensed under Creative Commons Attribution Share-Alike 2.5
• Hex board by Tiltec of Wikimedia Commons. Released into the public domain.
• Go-style hex board by Twixter of HexWiki. Licensed under Creative Commons Attribution Share-Alike 3.0.
• Blokus Trigon by Gangster 1930 of BoardGameGeek. Licensed under Creative Commons Attribution Non-Commercial Share-Alike 3.0.

Answer 2

One reason why the less natural hexagonal grid is so popular in wargames and other games where the board represents real-world terrain is that it measures distances much closer than the others. All six adjacent hexes are the same distance from the central hex, and the variations even out to four or five hexes are small. Both square and triangular grids have serious problems with unequal distances as a result of the diagonals.

If you're measuring the range of a gun by counting hexes then it won't be far off; if you're measuring on a square grid then it will be either 40% further or 30% less far along the diagonal. Variations on a hex grid are in single-digit percentages.

With a triangular grid is that you have the unnatural non-square grid without the advantages you get from the hex grid in not having any diagonals and having a more accurate representation of distance.

Answer 3

Another difference between the three grids is the following: for spaces that "touch", a square grid has two different relative positions: sharing an edge or touching diagonally. For hex grids, two spaces that "touch" can only share an edge. For a triangular grid, however, there are three options:

Consider the red triangle. It touches each of the three other non-white triangles; but:

• it shares an edge with the black triangle;
• it is "opposite" the green triangle (that is, you get the green triangle by mirroring the red triangle in one of it vertices);
• neither is true for the blue triangle.

By looking at the dual hex grid, we can see that the blue triangle is two steps away from the red triangle, whereas the black one is only one step away and the green one is three steps away.

Maybe you can use the different relationships that triangles can have to eachother, to your advantage when designing a game, by differentiating among them. In some sense, Settlers of Catan does that in some sense - it uses the triangular grid underlying the hex grid of the tiles for placing the cities and streets on, and streets can clearly only be used to connect "triangles that share an edge". However, cities whose triangles touch share a resource producing hexagon.

Answer 4

Humans tend to think on the rectangular grid. We've built our cities on the rectangular grid, and the primary coordinate system is on the rectangular grid. As a result, people don't know how to work with other systems very well.

Answer 5

Hopefully this isn't too long and rambling, but I'm writing this kind of "stream of consciousness" style...

• Triangular pieces would have lower surface area touching the board, which would make them somewhat more unstable unless they were shorter in height. I.e. easier to knock over.
• Shorter pieces may be more difficult to manipulate, since you have fewer free sides. Think of a Scrabble board - you can extract almost any piece without significantly affecting the pieces around it. This could be mitigated by providing a nub or grip sticking up from the piece to be grasped.
• As McKay mentioned, we tend to think in squares. Up, down, left and right are simple to grasp. How do you describe directions or positions on a triangular board?
• Perhaps not as big of an issue, but manufacturing - particularly at the prototype level - would require additional expertise. Just about anybody can easily cut square pieces, but equilateral triangles would require more effort to make.
• Games played on a hexagonal board are typically because of the increased freedom of movement. Triangles would actually constrain movement even more tightly than squares. So a triangular board may work best for "tile"-like games that don't require much movement of pieces once they are placed.
• What happens when you bump the board? On a square (or hexagonal) board, there are always two faces parallel to one another, so the pieces will slide in a straight line. Triangular pieces will push their way to one side or the other from the axis of movement.

Answer 6

One tiny note to add to the more than sufficient existing answers: When using circular pieces (or pieces with a circular base) a hexagonal board is a more efficient use of space. Hexagons are the shape with the largest number of sides (hence being closer to a circle) that tessalate.

Hence a triangular grid board with the same number of spaces would have to be larger than its hexagonal equivilent to accomodate the same size circular pieces.

Answer 7

One obvious reason: space on the card. Squares and hexes lend themselves to game text, while it can be tricky to fit a significant amount of game text on a triangle.

Answer 8

One thought I've had is that most games are 2 player and a triangular board doesn't lend itself to 'opposition' type games. But if a triangular grid were made into a rhombic or other shape that might help.

I counted 7 chess like piece moves on a triangular grid, though there may be more.