Largest cumulative poker equity swing

Question

I don't know the best way to phrase this question, so I'll apologize in advance if it seems a little confusing. I also don't know if it's more appropriate for poker.se or stats.se (or some other forum- please point me in the right direction if anyone knows a better place). I'm posing it here because the problem doesn't seem computationally viable and the intuition of a board/card gamer might be appropriate.

Assume we have two players, P1 and P2 holding some hole cards and playing Texas Hold-Em. These two pairs have some certain pre-flop equity against each other (representing the probability that each hand will win come showdown.) Without loss of generality, assume we will keep track of P1's equity in our experiment.

After the flop comes, there is some new equity for P1. Similarly after the turn, and after the river. Every combination of cards and runouts has a "total equity swing" which I'll define as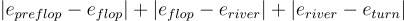

I'm wondering what the largest possible equity swing is across all pairs of hole cards and runouts is.

It's most likely that some one-outer will have to be hit on the river, and something close to a one-outer on the turn (if that even makes sense).

Answer 1

Strategically, let's aim for the worst relative starting position, then flip it completely at every opportunity. This matches the intuition of a one-outer following something close to it.

The best and worst hands respectively are pocket aces against seven-deuce offsuit. All percentages are given for the players in the same order, Player 1 (AA) versus Player 2 (7-2x).

Using the calculator at CardPlayer.com, the initial equity is 87.24% versus 12.40%.

With a flop of both deuces matching the aces and any offsuit three, we are now at 10.51% versus 89.49%.

Turn an ace to make a true rainbow, and it's back to 97.73% versus 2.27%.

Last deuce on the river and you get 0% versus 100% just to rub in the swing of this hand.

That gives equity swings of

• 76.73% and 77.09% from preflop to flop (Player 2 picks up the preflop tie chance of 0.36%)

• 87.22% both from flop to turn

• 97.73% both from turn to river

• 261.68% and 262.04% in total through the hand, or 523.72% equity swing on this hand.