In Gin-Rummy, in the start of a round players draw 10 cards.
What is the probability to draw 10 cards such that all the cards are part of some meld?
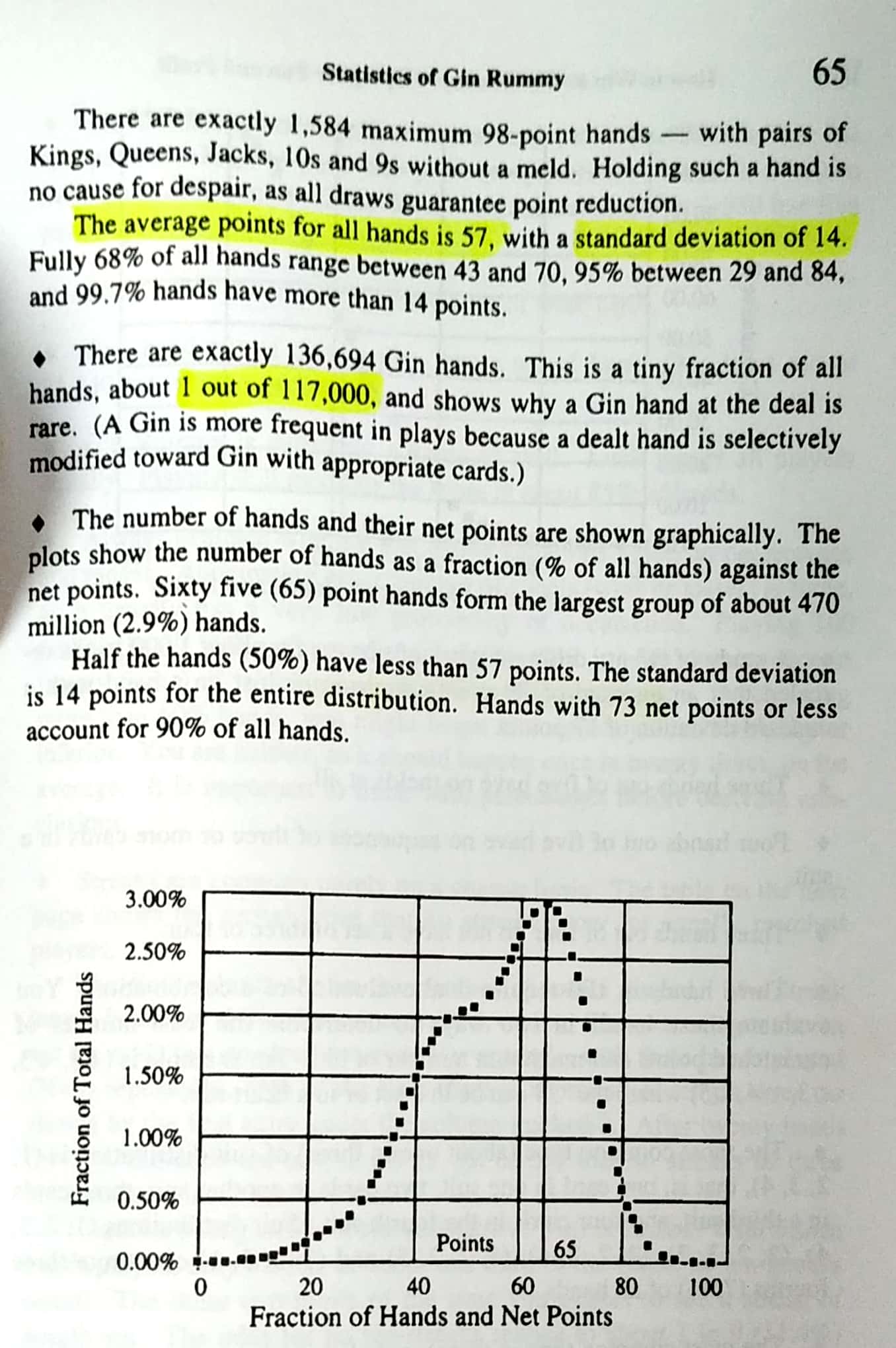
Three Independent sources counted about 136,694 Gin hands, out of 15.8B possible 10-cards hands.
2+2 forum post: 1 in ~118,000. Using brute force which checked for Gin each of the 15.8B hands.
Rulemonger's analysis: 136,694 in 15,820,024,220 or 1 in ~115733
How to Win at Gin Rummy: 1 in ~117,000, according to the book How to Win at Gin Rummy: Playing for Fun and Profit which state that there are 136,694 Gin hands out of 15,820,024,220 hands. See page 65:
EDIT: this count is flawed, it doesn't recognize sets of three that include a spade. The correct number is given in the accepted answer. When the code is corrected, it gives the 136,694 unique gin hands, matching the accepted answer.
Original:
1 in 308,984, according to Andrew Inwood's analysis, which includes source code.